Matching Theory to Characterize Sound Emission during Vortex Reconnection in Quantum Fluids
Abstract
In a concurrent work, Villois et al. [Phys. Rev. Lett. 125, 164501 (2020)] reported the evidence that vortex reconnections in quantum fluids follow an irreversible dynamics, namely, vortices separate faster than they approach; such time asymmetry is explained by using simple conservation arguments. In this work we develop further these theoretical considerations and provide a detailed study of the vortex reconnection process for all the possible geometrical configurations of the order parameter (superfluid) wave function. By matching the theoretical description of incompressible vortex filaments and the linear theory describing locally vortex reconnections, we determine quantitatively the linear momentum and energy exchanges between the incompressible (vortices) and the compressible (density waves) degrees of freedom of the superfluid. We show theoretically and corroborate numerically, why a unidirectional density pulse must be generated after the reconnection process and why only certain reconnecting angles, related to the rates of approach and separations, are allowed. Finally, some aspects concerning the conservation of center-line helicity during the reconnection process are discussed.
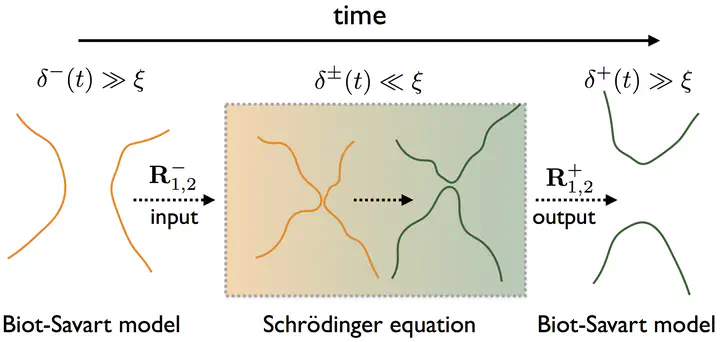
The figure above shows a sketch of a vortex reconnection process and how is theoretically handled. For a movie showing the full evolution of the vortices from a Gross-Pitaevskii simulation go the News section or click here!
This work extends the results presented in Villois et al. 2020. A Mathematica notebook with detailed calculations is provided as Supplemental Material.