Testing Wave Turbulence Theory for the Gross-Pitaevskii System
Physical Review E Editor’s Suggestion
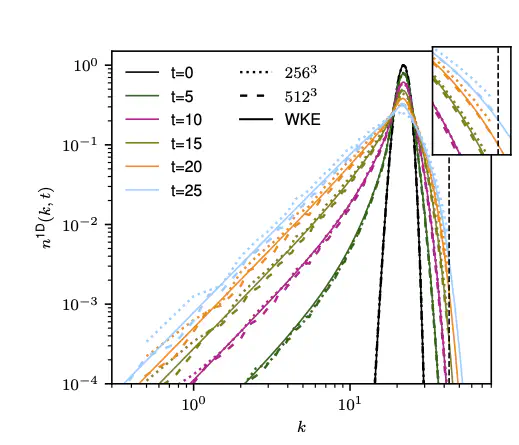 Numerical comparison between simulations of the Gross-Piteavskii equation and its associated wave kinetic equation.
Numerical comparison between simulations of the Gross-Piteavskii equation and its associated wave kinetic equation.
Abstract
We test the predictions of the theory of weak wave turbulence by performing numerical simulations of the Gross-Pitaevskii equation (GPE) and the associated wave-kinetic equation (WKE). We consider an initial state localized in Fourier space, and we confront the solutions of the WKE obtained numerically with GPE data for both, the wave action spectrum and the probability density functions (PDFs) of the Fourier mode intensities. We find that the temporal evolution of GP data is accurately predicted by the WKE, with no adjustable parameters, for about two nonlinear kinetic times. Qualitative agreement between the GPE and the WKE persists also for longer times with some quantitative deviations that may be attributed to the breakdown of the theoretical assumptions underlying the WKE. Furthermore, we study how the wave statistics evolves toward Gaussianity in a time scale of the order of the kinetic time. The excellent agreement between direct numerical simulations of the GPE and the WKE provides a new and solid ground to the theory of weak wave turbulence.